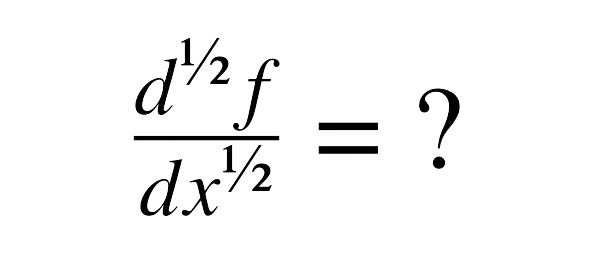
Continuity, most of the functions we study in elementary calculus are described by simple formulas. These functions almost always possess derivatives and, in fact, a portion of any first course in calculus is devoted to the development of routine methods for computing derivatives. However, not all functions possess derivatives everywhere. For example, the functions ![]() do not possess derivatives at x = 0 no matter how they are defined at x = 0.
do not possess derivatives at x = 0 no matter how they are defined at x = 0.
As we progress in the study of analysis, it is important to enlarge substantially the class of functions under examination. Functions which possess derivatives everywhere form a rather restricted class; extending this class to functions which are differentiable except at a few isolated points does not enlarge it greatly. We wish to investigate significantly larger classes of functions, and to do so we introduce the notion of a continuous functions.
Definitions
Suppose that ƒ is a function from a domain D in ![]() to
to ![]() . The function ƒ is continuous at a if and only if (i) the point a is in an open interval I contained in D, and (ii) for each positive number ε & there is a positive number δ such that
. The function ƒ is continuous at a if and only if (i) the point a is in an open interval I contained in D, and (ii) for each positive number ε & there is a positive number δ such that
![]()
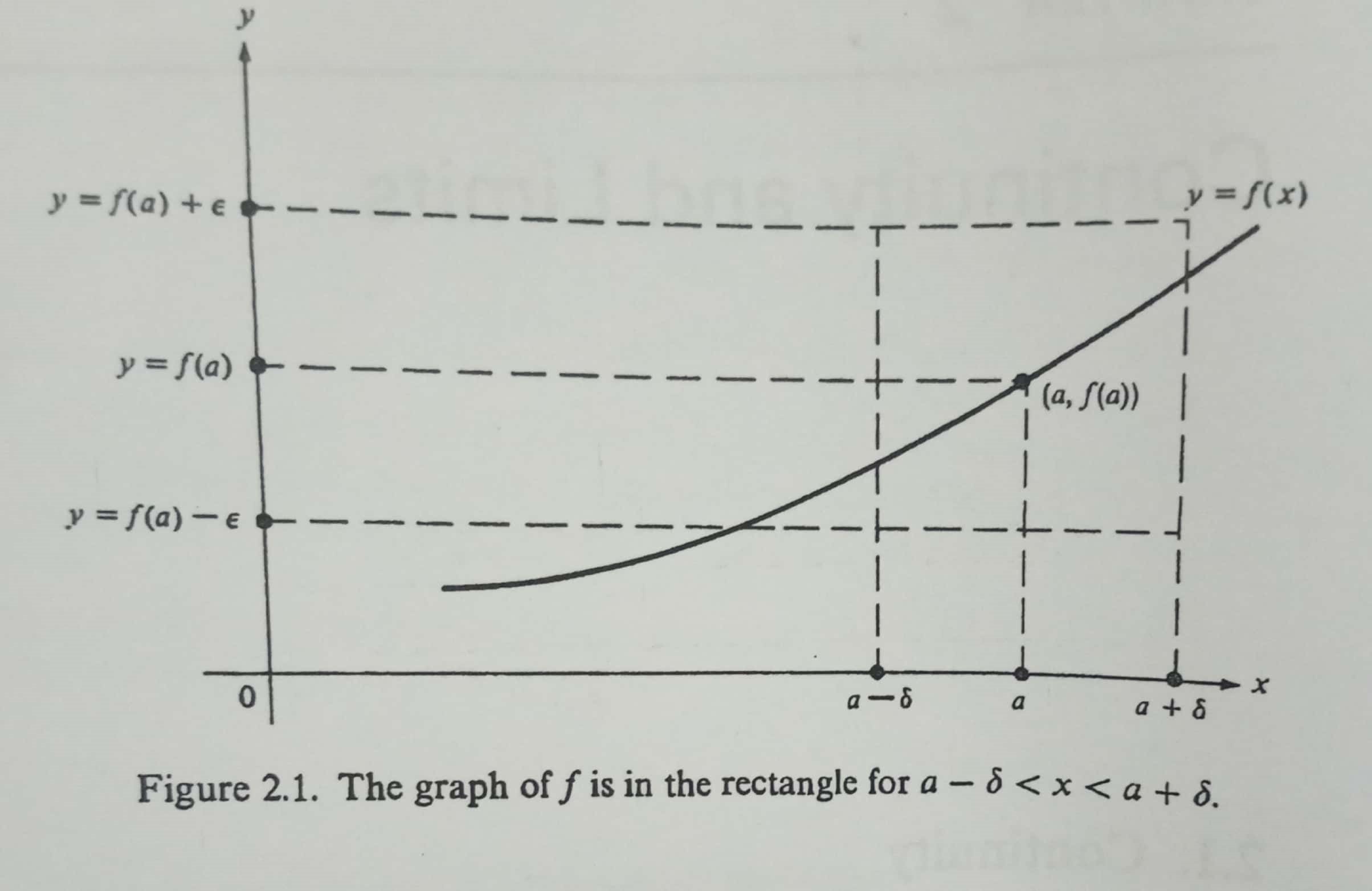
If f is continuous at each point of a set S, we say that ƒ is continuous on S. A function ƒ is called continuous if it is continuous at every point of its domain. The geometric significance of continuity at a point a is indicated in Figure 2.1. We recall that the inequality ![]() is equivalent to the double inequality
is equivalent to the double inequality
![]()
or,
![]()
Similarly, the inequality ![]() is equivalent to the two inequalities
is equivalent to the two inequalities
![]()
We construct the four lines ![]() as shown in Figure 2.1. The rectangle determined by these four lines has its center at the point with coordinates (a, f(a)). The geometric interpretation of continuity at a point may be given in terms of this rectangle. A function ƒ is continuous at a if for each
as shown in Figure 2.1. The rectangle determined by these four lines has its center at the point with coordinates (a, f(a)). The geometric interpretation of continuity at a point may be given in terms of this rectangle. A function ƒ is continuous at a if for each ![]() there is a number
there is a number ![]() such that the graph of ƒ remains within the rectangle for all x in the interval
such that the graph of ƒ remains within the rectangle for all x in the interval ![]() .
.
It is usually very difficult to verify continuity directly from the definition. Such verification requires that for every positive number ![]() , we exhibit a number
, we exhibit a number ![]() and show that the graph of ƒ lies in the appropriate rectangle. However, if the function f is given by a sufficiently simple expression, it is sometimes possible to obtain an explicit value for the quantity
and show that the graph of ƒ lies in the appropriate rectangle. However, if the function f is given by a sufficiently simple expression, it is sometimes possible to obtain an explicit value for the quantity ![]() corresponding to a given number
corresponding to a given number![]() . We describe the method by means of two examples.
. We describe the method by means of two examples.
Example 1.
Given the function
![]() ,
,
and ![]() , find a number
, find a number ![]() such that
such that
![]() for
for ![]() .
.

Figure 2.2
Solution
We sketch the graph of ƒ and observe that ƒ is decreasing for x > -1 (see Figure 2.2). The equations f(x) − f(1) = 0.1, f(x) – f(1) = -0.1 can be solved for x. We find
![]()
Since ƒ is decreasing in the interval ![]() , it is clear that the graph of ƒ lies in the rectangle formed by the lines
, it is clear that the graph of ƒ lies in the rectangle formed by the lines ![]() . Since the distance from x = 1 to x = 2/3 is smaller than the distance from x = 1 to x = 3/2, we select
. Since the distance from x = 1 to x = 2/3 is smaller than the distance from x = 1 to x = 3/2, we select ![]() . We make the important general observation that when a value of
. We make the important general observation that when a value of ![]() is obtained for a given quantity ε, then any smaller (positive) value for
is obtained for a given quantity ε, then any smaller (positive) value for ![]() may also be used for the same number ε.
may also be used for the same number ε.
Remarks.
For purposes of illustration, we assume in this chapter that ![]() is defined for all
is defined for all ![]() if n is even and that
if n is even and that ![]() is defined for all x if n is odd. We also suppose that for positive numbers
is defined for all x if n is odd. We also suppose that for positive numbers ![]() , the inequality
, the inequality ![]() holds whenever
holds whenever ![]() . Facts of this type concerning functions of the form
. Facts of this type concerning functions of the form ![]() , with n a natural number, do not follow from the results given thus far.
, with n a natural number, do not follow from the results given thus far.

Figure 2.3
Example 2.
Consider the function
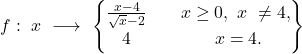
If ![]() = 0.01, find a
= 0.01, find a ![]() such that
such that ![]() for all x such that
for all x such that ![]() .
.
Solution
If ![]() , then
, then
![]()
The graph of ƒ is shown in Figure 2.3, and we observe that ƒ is an increasing function. We solve the equations f(x) – f(4) = 0.01 and f(x) − f(4) = -0.01 and obtain
![]()
![]()
Since ƒ is increasing, it follows that ![]() for 3.9601 <x< 4.0401. Selecting
for 3.9601 <x< 4.0401. Selecting ![]() = 0.0399, we find that
= 0.0399, we find that ![]() for
for ![]() .
.
We shall frequently be concerned with functions which have a domain in ![]() which consists of an interval except for a single point. This exceptional point may be an interior point or an endpoint of the interval. For example, the function
which consists of an interval except for a single point. This exceptional point may be an interior point or an endpoint of the interval. For example, the function ![]() is defined for all x except x = 0. The function log x is defined for x>0 but not for x = 0. Other examples are cot x, defined on any interval not containing an integral multiple of
is defined for all x except x = 0. The function log x is defined for x>0 but not for x = 0. Other examples are cot x, defined on any interval not containing an integral multiple of ![]() , and the function
, and the function ![]() not defined for x = -2; in fact, any function defined as the quotient of two polynomials has excluded from its domain those values at which the denominator is zero.
not defined for x = -2; in fact, any function defined as the quotient of two polynomials has excluded from its domain those values at which the denominator is zero.
Definition
Suppose that a and L are real numbers and ƒ is a function from a domain D in ![]() to
to ![]() . The number a may or may not be in the domain of f. The function f tends to L as a limit as x tends to a if and only if (i) there is an open interval I containing a which, except possibly for the point a, is contained in D, and (ii) for each positive number
. The number a may or may not be in the domain of f. The function f tends to L as a limit as x tends to a if and only if (i) there is an open interval I containing a which, except possibly for the point a, is contained in D, and (ii) for each positive number ![]() there is a positive number
there is a positive number ![]() such that
such that
![]() whenever
whenever ![]()
If ƒ tends to L as x tends to a, we write
![]() as
as ![]()
and denote the number L by
![]()
or by
![]()
Remarks
(i) We see that a function f is continuous at a if and only if a is in the domain of ƒ and ![]() as
as ![]() .
.
(ii) The condition ![]() (excluding the possibility x = a) is used rather than the condition
(excluding the possibility x = a) is used rather than the condition ![]() as in the definition of continuity since f may not be defined at a itself.
as in the definition of continuity since f may not be defined at a itself.